A ball swings counterclockwise in a vertical circle, a captivating phenomenon that showcases the interplay of forces and motion. This intricate dance of physics unfolds as the ball traces a circular path, suspended by a string, inviting us to delve into the underlying principles that govern its trajectory.
As the ball embarks on its circular journey, centripetal force takes center stage, acting as the invisible hand that keeps it tethered to the center of the circle. Simultaneously, the tension in the string plays a crucial role, providing the necessary centripetal force to maintain the ball’s circular motion.
The interplay of these forces orchestrates the ball’s graceful ascent and descent, revealing the delicate balance that underpins its motion.
Centripetal Force
A ball swinging counterclockwise in a vertical circle experiences a centripetal force that keeps it moving in a circular path. This force is directed towards the center of the circle and is provided by the tension in the string attached to the ball.
Direction and Magnitude
The direction of the centripetal force is always perpendicular to the ball’s velocity vector and points towards the center of the circle. The magnitude of the centripetal force is given by:
Fc= m
v2/ r
where m is the mass of the ball, v is its speed, and r is the radius of the circle.
Tension in the String
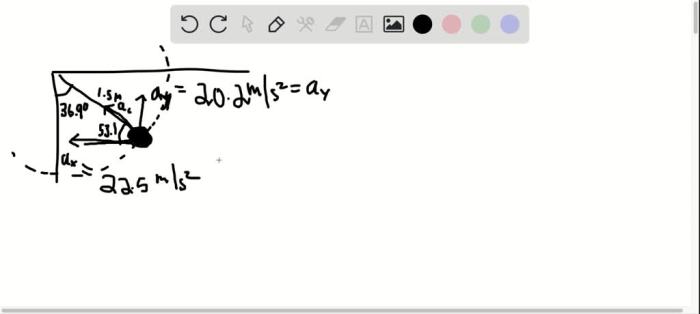
The string attached to the ball plays a crucial role in maintaining the ball’s circular motion. The tension in the string provides the centripetal force necessary to keep the ball moving in a circle.
Variation with Ball’s Position
The tension in the string varies with the ball’s position in the circle. It is maximum at the top of the circle, where the ball is moving slowest, and minimum at the bottom of the circle, where the ball is moving fastest.
Energy Considerations
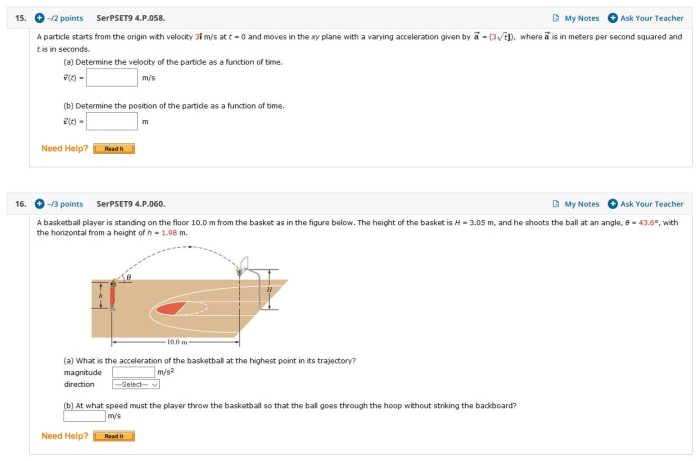
The ball’s motion in a vertical circle involves different forms of energy.
Forms of Energy, A ball swings counterclockwise in a vertical circle
- Kinetic energy: Due to the ball’s motion.
- Gravitational potential energy: Due to the ball’s height above the ground.
Conservation of Energy
The total energy of the ball remains constant throughout its trajectory, as long as there are no external forces acting on it.
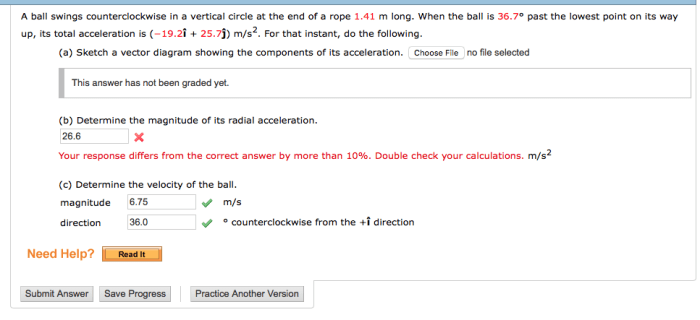
Circular Motion and Velocity: A Ball Swings Counterclockwise In A Vertical Circle
The ball’s velocity vector changes direction as it moves in a circle, but its magnitude remains constant.
Velocity Vector
At any point in its circular path, the ball’s velocity vector is tangent to the circle at that point.
Centripetal Acceleration
The ball’s velocity vector changes direction, so it experiences a centripetal acceleration that is always perpendicular to its velocity vector and directed towards the center of the circle.
Angular Displacement and Frequency
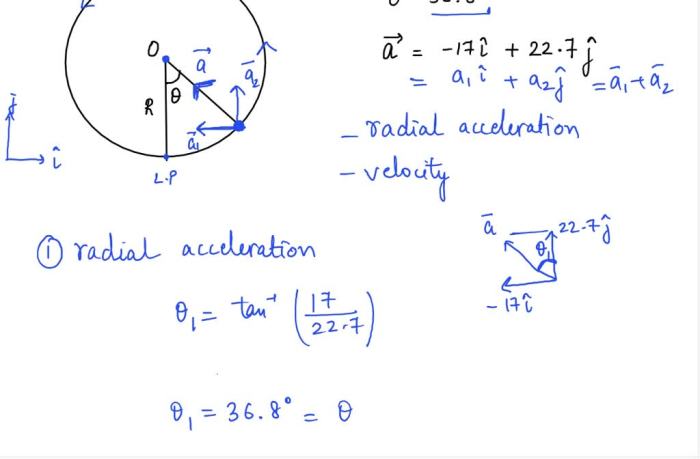
The ball’s angular displacement and frequency describe its motion in terms of angles.
Angular Displacement
Angular displacement is the angle through which the ball rotates, measured in radians.
Angular Frequency
Angular frequency is the rate at which the ball rotates, measured in radians per second.
Calculation
The angular displacement and frequency can be calculated using the following equations:
θ = ω
t
ω = 2πf
where θ is the angular displacement, ω is the angular frequency, t is the time, and f is the frequency.
Questions and Answers
What is the direction of the centripetal force acting on the ball?
The centripetal force is always directed towards the center of the circle.
How does the tension in the string vary with the ball’s position in the circle?
The tension in the string is greatest when the ball is at the bottom of the circle and least when the ball is at the top of the circle.
What is the relationship between the ball’s velocity and its centripetal acceleration?
The ball’s velocity is always perpendicular to its centripetal acceleration.